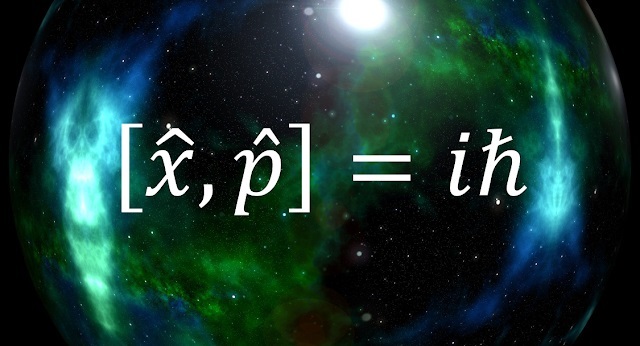交換子
量子力学においては,物理量は波動関数に作用する演算子によって表されることを議論してきた。
二つの物理量に対応する演算子$\hat{A}$と$\hat{B}$を考え,それらの固有値をそれぞれ$a_n$と$b_n$で表す。
波動関数$\psi_n$が双方の演算子の固有関数であったなら,それらを続けて演算した結果は
\begin{equation}
\hat{A}\hat{B}\psi_n
=\hat{A}(b_n\psi_n)
=a_n b_n \psi_n
\end{equation}
となる。
この結果は,演算子を作用する順番を入れ替えても成り立つ。
この性質は,二つの演算子$\hat{A}$と$\hat{B}$に対し
\begin{equation}
\label{eq:commutator_AB}
[\hat{A},\hat{B}]
=
\hat{A}\hat{B}-\hat{B}\hat{A}
\end{equation}
を定義すると
\begin{equation}
[\hat{A},\hat{B}]
=0
\end{equation}
と表せる。
任意の演算子に対し,(\ref{eq:commutator_AB})を交換子(commutator)と呼び,交換子を用いて与えられる関係を,交換関係(commutation relation)と呼ぶ。
そして,交換子がゼロとなる場合,それらの演算子は交換(commute)するとか,可換(commutative)であるという。
今考えたのは,二つの演算子$\hat{A}$と$\hat{B}$が同一の固有関数を持ち,それぞれの固有値が得られる場合である。
物理的には,対応する物理量が同時に確定した値を持つことを意味している。
正準交換関係
一般には二つの演算子は可換とは限らない。
運動量演算子と位置演算子の交換関係を考えてみよう。
簡単のために1次元で考える。
古典力学では,$(xp-px)=0$であるが,量子力学の場合,運動量演算子は関数$\psi(x)$だけなく座標$x$にもひっかかるため
\begin{equation}
\begin{split}
(\hat{x}\hat{p}-\hat{p}\hat{x})\psi(x) \\
=& -i\hbar\left(x\frac{\partial \psi}{\partial x}-\frac{\partial(x\psi)}{\partial x} \right) \\
=& -i\hbar \left( x\frac{\partial \psi}{\partial x}-x\frac{\partial\psi}{\partial x}-\psi \right) \\
=& i\hbar \psi(x)
\end{split}
\end{equation}
となる。
したがって,交換子を用いると
\begin{equation}
[\hat{x},\hat{p}]=i\hbar
\end{equation}
である。
位置と運動量は,Hamilton力学では正準共役と呼ばれる関係にある変数であり,対応する演算子が交換子の演算の下で満たす関係を一般に正準交換関係(canonical commutation relation)という。
3次元への拡張は容易で
\begin{align}
[\hat{x}_i,\hat{p}_j]=i\hbar \delta_{ij}, \ \ i,j=1,2,3
\end{align}
となることが確かめられる。
ここで$\delta_{ij}$はKroneckerのデルタで,$i=j$のときに1となり,それ以外はすべて0となる。
いくつかの関係
後のために,正準交換関係を用いていくつかの関係を調べておこう。
Hamiltonianと位置演算子の交換関係は
\begin{equation}
\begin{split}
\left[\hat{H},\hat{x}\right]
=&\left[\frac{ \hat{p}^2}{2m}+V,\hat{x}\right] \\
=&\frac{1}{2m}\left[{\hat{p}}^2,\hat{x}\right] \\
=&\frac{1}{2m} \left( \hat{p} \left[ \hat{p},\hat{x} \right] + \left[ \hat{p},\hat{x} \right] \hat{p} \right) \\
&=-\frac{i\hbar}{m} \hat{p}
\end{split}
\end{equation}
Hamiltonianと運動量の交換関係は
\begin{equation}
\begin{split}
\left[\hat{H},\hat{p}\right]
=&
\left[\frac{{\hat{p}}^2}{2m}+V,\hat{p}\right] \\
%
=&
V\left(-i\hbar\frac{\partial}{\partial x}\right)-\left(-i\hbar\frac{\partial}{\partial x}V\right) \\
%
=&
i\hbar\frac{\partial}{\partial x}V
\end{split}
\end{equation}
と計算される。